If you wondering what are coefficients in mathematics , this blog helps explain what they are,how they shape algebraic expressions, and their crucial role in solving equations.Coefficients stand as pillars that support the structure of various mathematical expressions and equations. A strong understanding of coefficients is essential whether you are a student navigating the challenges of algebra or an experienced mathematician investigating advanced calculus. This blog explores the types, meaning, and practical uses of coefficients in order to shed light on their mysterious nature.
Learnerscamp is a dedicated platform designed to assist learners in comprehensively understanding the concept of coefficients.Through practical questions and clear explanations, Learnerscamp provides the essential resources to help you grasp the fundamentals of what are coefficients in mathematics and get started on your learning journey.
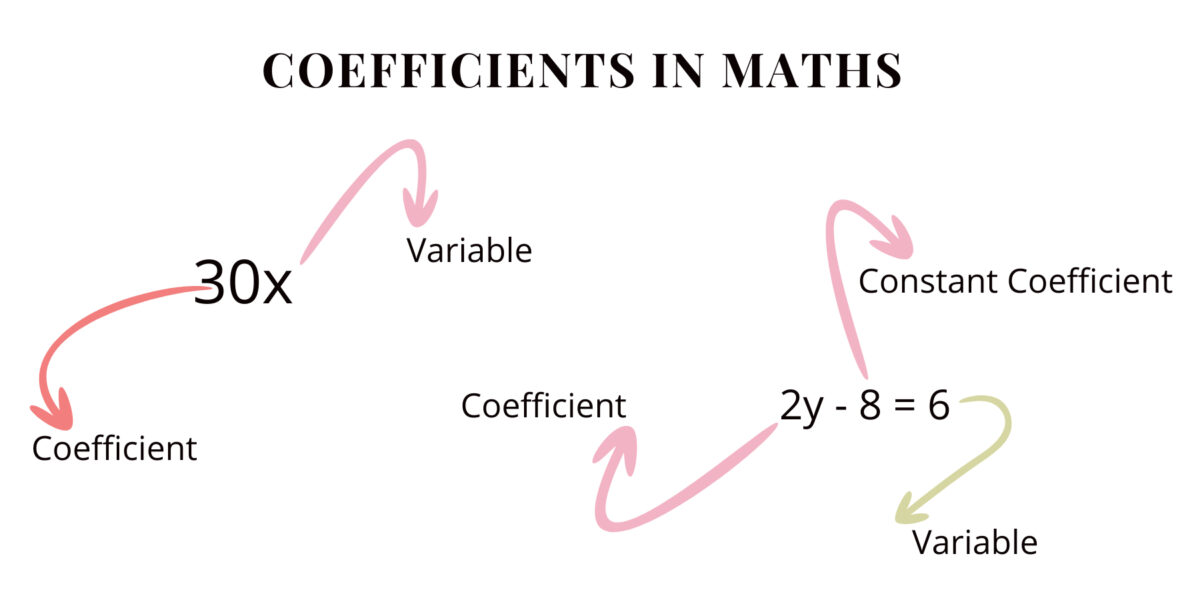
What Are Coefficients in Mathematics?
At its core, a coefficient is a numerical or constant factor that accompanies and multiplies a variable in an algebraic expression. Let’s break this down with a simple example: consider the expression 3x. Here, 3 is the coefficient, and x is the variable. The coefficient tells us how many times the variable is being multiplied.
Coefficients are the fundamental building blocks of mathematical expressions; they are numerical constants that interact with variables to give equations depth. Determining the value and significance of each term in an equation is largely dependent on coefficients, which are like keys to the language of mathematics.
Exploring the Types of Coefficients:
Numeric Coefficients:
Numeric coefficients are constants in front of variables in algebraic expressions. They indicate the number by which the variable is multiplied. Numeric coefficients embody simplicity. Consider the following examples
Example 1: 5x
5 is the numeric coefficient determining the multiplication factor of the variable x.” .It implies that the variable x is multiplied by 5.
The expression 5x can be interpreted as “five times x”
Example 2: -2y
In this case,the numeric coefficient is -2.The negative sign indicates that the variable y is multiplied by -2.
The expression −2y can be understood as “negative two times y.”
Literal Coefficients
Moving beyond simplicity, literal coefficients involves variables alongside numerical constants. Picture the expressions below:
Example 1: 2ab
2ab, where 2 is the numeric coefficient, and ab forms the literal coefficient. Example 2: a.x In this expression, a is the literal coefficient.It indicates that the variable x is multiplied by the variable a.
The expression a⋅x can be interpreted as “the product of a and x.”
Literal coefficients are versatile because they allow for the representation of general relationships where the specific values of variables are not known. These coefficients are prevalent in mathematical modeling and various scientific disciplines, providing a flexible way to express relationships between different quantities.
Constant Coefficients
Constants, devoid of any accompanying variables, stand independently in equations.
Consider the equation 7 = 2x + 5, where both 2 and 5 serve as constant coefficients.
Significance of Coefficients:
Coefficients are not mere abstract entities; they hold significant implications across various mathematical realms:
- Equations and Inequalities: Coefficients govern the relationships between variables and constants, offering a pathway to solve intricate equations and inequalities.
- Polynomials: In polynomial expressions, coefficients guide the degree and behavior of each term, shaping the overall properties of the polynomial.
- Linear Algebra: The significance of coefficients extends to systems of linear equations, where matrices and vectors represent these numerical constants and variables.
Coefficients in Real-world Applications
Extend your understanding beyond textbooks and classrooms:
Physics
Coefficients find their place in physical equations, influencing factors such as friction, resistance, and elasticity. They become the language through which physical phenomena are described and analyzed.
Engineering
Engineers rely on coefficients to model and analyze diverse systems, from electrical circuits to structural designs. Coefficients become the bridge between mathematical abstraction and real-world applications.
As we traverse the applications of mathematical coefficients, their integral role becomes evident. From solving algebraic equations to modeling complex systems in engineering, a profound understanding of coefficients in mathematics becomes the guiding light.
By now you should be able to answer the question What are Coefficients in Mathematics, this exploration into the significance and applications of coefficients in mathematics equips us with a deeper understanding of the language that underpins the mathematical universe. Remember, as you encounter algebraic expressions or delve into the complexities of engineering systems, it’s the coefficients that hold the key to unraveling the mysteries of mathematics.